Calibration of AFM Cantilevers

 

An important application of the atomic force microscope is in quantitative force measurements, a prerequisite of which is accurate knowledge of the cantilever spring constant. Although several calibration methods have been described in the literature, one must often wade through numerous publications in order to track down all of the details. Here, we briefly review three popular methods and single out one — the thermal noise method — for a detailed description. If all you are looking for is the method, feel free to skip to Section 2.

1. Calibration Methods

A number of ways to calibrate AFM cantilevers have been described in the literature. Methods employed have included:

• theoretical calculations based on cantilever dimensions and materials properties (often based on a rectangular beam approximation)
• measurement of the gravitational deflection due to added masses
• measurement of the deflection due to cantilevers of known spring constant
• measurement of the deflection due to the viscosity of the medium surrounding the cantilever

Fairly complete reviews can be found in the literature. See for example Refs. [1,2].

It is worth noting that the nominal value of the spring constant quoted by the manufacturer is often based on the first approach and is therefore sensitive to defects and batch-to-batch variations (though quoting conservative ranges of values is now common). Deviations from claimed values are often as high as a factor of two, demonstrating the need for independent calibrations.

Perhaps the most accessible calibration methods are calibration against known references [3], the Cleveland method [4], and the thermal noise method [5].

Calibration against a known standard:

In this method, a lever of known spring constant is attached to a sample substrate and the AFM cantilever is deflected by performing a force curve at the end of the lever. By noting the slope of the force curve relative to that obtained on a rigid substrate (or, better yet, relative to a series of different known levers), the spring constant of the cantilever can be deduced.

In a force curve, the A–B (deflection signal) is plotted against piezo extension z. In general, the A–B signal is uncalibrated and will be different for every cantilever used. Therefore, we usually measure the deflection in arbitrary units (i.e., Volts), requiring that a detector calibration factor s be found such that the actual cantilever deflection from equilibrium, y = sV, where V is the deflection signal relative to the equilibrium cantilever deflection.

It is easy to show that the slope dV/dz is related to the spring constants of the cantilever k₀ and reference lever K according to

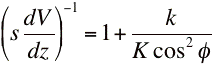
Note that the angle ø — the tilt angle of the cantilever — has until recently been neglected [6]. The corresponding factor arises because only a cosø component of the vertical force due to the reference lever acts to deflect the cantilever, and only a cosø component of that deflection is along the measured vertical direction. In practice, this correction is typically a few percent, which is less than the uncertainty in the method.

Therefore, if a series of reference levers is employed, the cantilever spring constant is determined simply from the slope m and intercept b of a plot of force-curve slopes dV/dz arising from each reference-lever spring constant K in the series. We find

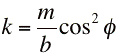
Difficulties with this approach include the fact that reference levers with spring constants near that of the cantilever to be calibrated must be available. Care must be taken to position the AFM cantilever so that it contacts the end of the reference lever (the fractional error in position leads to a cubic correction). It is obviously also essential that the spring constant of the reference levers be known. In one case (unpublished), we found that a series of calibrations performed using this method gave a result that disagreed by a factor of nearly two with both the Cleveland and thermal noise methods (which agreed well with each other), suggesting that the "accurately known" standards were in error. Nevertheless, this is a very direct method if care is taken.

The Cleveland Added-Mass Method:

The Cleveland method determines the cantilever spring constant from the decrease in resonant frequency resulting from the attachment of known masses. The theory is very simple: one expects the resonant frequency f to depend on added mass M according to

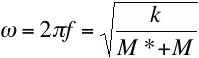
where M* is the effective mass of the cantilever. Thus, if a series of different masses are attached, a plot of M vs. 1/f² yields a straight line, the slope m of which can be used to determine the spring constant:

This method can be employed even without a micromanipulator. We use the coarse positioning screws on the AFM head to move the cantilever into contact with tungsten spheres (used because of their high density) deposited on a clean, dry surface. After picking up a sphere (a somewhat tedious task), we measure the new resonant frequency (which can typically be done with built-in hardware for AFMs capable of intermittent contact mode or other AC imaging techniques). We then remove the tip holder, invert it, and carry it to an optical microscope, which is used to take a high-magnification picture of the cantilever and attached mass. We estimate the mass of the tungsten sphere from its diameter and known density. This process is repeated until either a sufficient number of different resonant frequencies have been recorded, the cantilever is lost or broken, or the operator gets bored.

An important detail is that because the further the mass M is from the base of the cantilever, the more it will lower the resonant frequency, its mass must be corrected according to its position relative to the cantilever tip [7]:

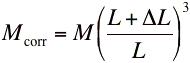
The main disadvantages of this method are that it is somewhat time intensive (even with the apparatus and a substrate of tungsten spheres in place, spheres must be picked up by the cantilever and imaged optically) and destroys the cantilever (even if excessive force is avoided during the procedure, one is generally left with a cantilever littered with tungsten spheres at the end of the day). The significant advantage that resonant frequencies are accurately measured is offset, at least partially, by uncertainties in the measurement of position and size of the added masses, both of which enter the calculations cubically.

The Thermal Noise Method:

The thermal noise method appeals to the equipartition theorem, which states that the thermal energy present in all terms in the Hamiltonian of a system that are quadratically dependent on a generalized coordinate is equal to k_BT/2, where k_B is Boltzmann's constant and T is the absolute temperature (in Kelvin). If one can treat the cantilever as an ideal spring of constant k, a measurement of the thermal noise <x²> in its position allows the spring constant to be determined as

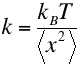
Unfortunately, the cantilever cannot be treated as an ideal spring. A series of improvements, discussed in Section 3 below, lead to the correct calibration formula

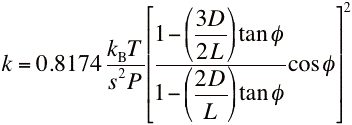
where

• the numerical factor 0.8174 is a result of the geometry of the cantilever spring
• s is again the sensitivity calibration factor (in units of m/V)
• P is the positional noise power (in units of V²) isolated in the fundamental resonant mode only
• D is the height of the tip
• L is the cantilever length (more correctly, the distance from the base of the cantilever to the tip

and the term in square brackets accounts for the cantilever tilt relative to the substrate and the geometry of the optical-lever detection scheme commonly used in commercial AFMs. This "thermal calibration formula," first presented in Ref. [6] will be used in the discussion below.

The thermal noise method is limited by the sensitivity of the device used to measure the noise in the deflection signal: stiffer cantilevers suffer less thermal vibration. One source of error is the calibration factor s, which can be obtained from a force curve against a rigid substrate. In many cases an oscillatory baseline due to interference between the reflected laser beam and laser light scattered from the substrate can lead to an incorrect estimate of s (the calibration against a known standard is also succeptible to such errors).

The primary advantage of the thermal noise method is ease of use. Once the apparatus and procedures are in place, it takes approximately 5 minutes to calibrate a cantilever, including mounting the cantilever, measuring data, and analyzing the results.

2. The Thermal Noise Method: A Cookbook Approach

This section is meant as an aid to newcomers to AFM cantilever calibrations; here, I detail the method in as simple a way possible.

Equipment:

We use the following equipment in our lab:

1. atomic force microscope
   • ours is a Digital Instruments MultiMode with Nanoscope IIIa software
2. some method to acquire noise data (such as a high-speed ADC card or frequency analyzer)
   • we have access to the A–B deflection signal through a breakout box
   • we acquire data with a National Instruments NI 6052E, a 333 kHz, 16 bit ADC card
3. analysis software
   • we use Igor Pro 4 (Wavemetrics)
   • interfacing with the ADC card is through the Igor Pro NIDAQ package
   • the Igor macros we use will be available here

Note that the thermal calibration formula assumes an optical-lever deflection system (the most common for commercial instruments). If you are using something else, you will need to delve into the theory a bit to make it work for your case.

Measurements:

Our procedure is as follows:

1. mount a flat substrate in the AFM
   • clean silicon wafers or freshly-cleaved mica or graphite work well
2. mount cantilever in AFM
3. position laser for strong signal with compact spot size
   • signal measured with the AFM's A+B meter
   • spot inspected by inserting a small piece of paper in the beam
4. zero the A–B signal
   • achieved by positioning the photodetector in our instrument
5. do a coarse approach
6. do auto approach to sample
7. go to force curves
8. record A–B (deflection) data during force curves using ADC card
   • one cycle is enough
   • note ramp size and rate
9. withdraw from the substrate
   • by several microns, beyond the range of surface forces
10. record A–B (deflection) data at highest sensitivity
    • A–B signal must be zeroed well enough to make use of this sensitivity
    • data rate must be comfortably more than double the cantilever resonant frequency to be able to characterize the resonant peak
    • enough data should be acquired to allow a clean power spectrum to be generated (we usually record a time series of several million data points over several 10s of seconds)

These steps should be easily adaptable to other instruments.

Analysis

The data analysis (which we automate in Igor Pro) involves the following steps:

1. plot the force curve vs. piezo position
   • this is why you needed to know the ramp size and rate — the ADC card time-stamps the data in seconds, whereas we need a position in units of m
2. measure the slope of the contact portion of the force curve
   • a smallish deflection range should be used to avoid detector nonlinearities
   • since the substrate was rigid, the calibrated slope should be 1; thus the reciprocal of this slope is the deflection calibration factor s
3. calculate the power spectral density (PSD) of the noise data
   • this requires some care to get all of the factors of 2, etc., correct—if in doubt, see Numerical Recipes
   • better yet, test your algorithm with some fake data
   • the units will typically be V² before calibration
4. identify the peak corresponding to the fundamental resonant mode
   • the manufacturer's specs are usually enough to figure out which peak is which
   • beware of aliasing: if you suspect that a peak is not in the right place, try increasing your acquisition rate
   • also beware of electronic noise (usually a very sharp peak) or other vibrational modes
5. fit a Lorentizian (with baseline) to the peak
   • functional form is:
   
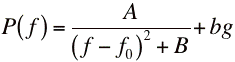
6. determine the area under the peak from the fit parameters
   
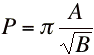
   • depending on how the PSD is defined in your particular algorithm, you may need additional factors such as 2, and the frequency bin size
   • in our case, we need to multiply by the number of points in our power spectrum
7. estimate the cantilever parameters D and L as well as the cantilever tilt angle
   • in most cases, the term in the thermal calibration formula in square brackets is close to unity, so these will not be greatly important
   • except in extreme cases, the manufacturer's specs will do
8. estimate the ambient temperature T of the cantilever
   • ours runs at about 305 K
9. apply the thermal calibration formula to determine k
   

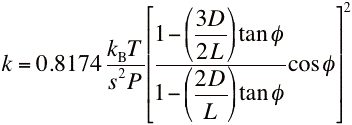
Our analysis routines also output an uncertainty. Typically, this is 5–10%, which is consistent with the agreement we typically see with the Cleveland added-mass method.

Advice

In critical cases, we typically calibrate our cantilevers using the thermal noise method at the beginning of an experiment, and at intervals throughout. Once we are convinced we no longer need the cantilever, we may perform an added-mass calibration.

Note that all three calibration methods discussed determine, at least in the formulations stated above, the intrinsic spring constant of the AFM cantilever — i.e., the spring constant that characterizes its perpendicular deflection resulting from the application of perpendicular forces. In real life, forces exerted by horizontal samples do not act in a direction perpendicular to the tilted cantilever, so measured forces must also be corrected from the naive expectation of F = ky. This seems a reasonable price to pay for using a definition of "spring constant" that does not depend on how the cantilever is mounted.

3. Thermal Noise Method: Theory and Background

This section presents a guided tour through the history of the thermal noise method for those who feel the need to work their way through the details.

The thermal noise method for calibrating AFM cantilevers dates back to 1993 [5]. As presented, it used the equipartition theorem to relate the measured thermal noise in the AFM cantilever deflection signal to the spring constant in the cantilever, which was assumed to behave like an ideal spring. In order to exclude other sources of noise, such as electronic noise, 60 Hz noise, table vibrations, etc., the authors recommended that the noise be measured in the frequency domain.

Butt and Jaschke [8] recognized that because a cantilever spring is not an ideal Hookean spring, its potential energy is not simply kx²/2. In order to relate the cantilever spring constant to the noise in its end position, one must express the Hamiltonian of the cantilever spring in terms of its normal modes. Moreover, the same authors pointed out that AFMs that use the optical-lever technique to sense the cantilever deflection actually measure the end slope of the cantilever as that is the quantity that determines the position of the reflected laster spot. The end slope is related to the end deflection, but the relationship is different for a cantilever who's end is supported by a surface (the configuration used to calibrate the deflection sensitivity) and one who's end is free to oscillate (the configuration in which the thermal noise is measured). The authors calculated a correction factor which also included the thermal noise in all cantilever vibrational modes, despite the technical difficulty (there is no way to discriminate against instrumental noise) and, indeed, the impossibility of obtaining such data (no instrument has infinite band width).

Walters, et al. [9] were apparently the first to note the need to limit the result of Butt and Jaschke to include the contribution of the fundamental vibrational mode only. When this is done, the original result of Ref. [5] needs to be modified by a numerical factor of 0.8174. This allowed them to calibrate cantilevers from the area of the fundamental resonant peak in power spectra of the vibrational noise. Since then, occasional claims have been made (by others) that overlap of this peak with the second vibrational mode can be significant; however, we have not seen this and believe that, except perhaps in cases with very broad resonances, this will not be important.

Spurred by an article in which Heim, et al. considered the effects of cantilever tilt on its response to forces, this author revisited the issue of calibration by thermal noise and obtained the correction for cantilever tilt and aspect ratio D/L included in the "final" thermal calibration formula presented here, as well as for the other two methods we have discussed in detail here [6]. Physically, cantilever tilt (which is necessary in all mounting systems) results is a cosine-squared correction: only a component of vertical forces exerted by the surface acts to deflect the cantilever, and only a component of that deflection is resolved along the vertical axis. The cantilever aspect ratio also results in a small correction. Although these corrections are small in typical cases, they become more important for the high-aspect ratio cantilevers favoured for high-resolution studies, and similar considerations (also discussed in Ref. [6]) can be important for colloidal probes.

It is worth noting that both the reference lever and added noise methods calibrate the deflection sensitivity by bending the cantilever with vertical forces exerted by a flat substrate. Therefore, the tilt effect was entirely compensated in previous measurements that employed either of those calibration schemes, leaving a much weaker aspect-ratio correction. This author recommends that, in future work, the intrinsic cantilever spring constant (i.e., tilt-independent response of the cantilever to perpendicular forces) be quoted, and measured forces be corrected for the cantilever tilt (and for the aspect-ratio in cases where it makes a significant difference). Details of this correction (which, again, is often small) are provided in Ref. [6].

Is this the last word on the thermal noise method? Perhaps not — implicit in the numerical corrections to the thermal noise result (as well as to force measurements in general, irrespective of the calibration method employed) is the assumption of a rectangular cantilever. Although many more rectangular cantilevers are commercially available now than were a decade ago, force data is often acquired with triangular cantilevers, which have different bending modes. Fortunately, numerical simulations of such cantilevers predict only minor corrections [11] so this is unlikely to be a concern until cantilevers can be calibrated to the 1% level rather than the current state-of-the-art of only 5-10%.

References

1. N.A. Burnham, et al., "Comparison of calibration methods for atomic-force microscopy canilevers." Nanotechnol. 14, 1–6 (2003).
2. C.T. Gibson, G.S. Watson, and S. Myhra, "Scanning force microscopy—calibrative procedures for 'best practice.'" Scanning 19, 564–581 (1997).
3. A. Torii, et al., "A method for determining the spring constant of cantilevers for atomic force microscopy." Meas. Sci. Technol. 7, 179–184 (1996).
4. J.P. Cleveland, et al. "A nondestructive method for determining the spring constant of cantilevers for scanning force microscopy." Rev. Sci. Instrum. 64, 403–405 (1995).
5. J.L. Hutter and J. Bechhoefer, "Calibration of atomic-force microscope tips." Rev. Sci. Instrum. 64, 1868–1873 (1993).
6. J.L. Hutter, "Comment on 'Tilt of atomic force microscope cantilevers: effect on spring constant and adhesion measurements." Langmuir 21, 2630–2632 (2005).
7. J.E. Sader, et al. "Method for the calibration of atomic force microscope cantilevers." Rev. Sci. Instrum. 66, 3789–3798 (1995).
8. H.-J. Butt and M. Jaschke, "Calculation of thermal noise in atomic force microscopy." Nanotechnol. 6, 1–7 (1995).
9. D.A. Walters, et al. "Short cantilevers for atomic force microscopy." Rev. Sci. Instrum. 67, 2580–3590 (1996).
10. L.-O. Heim, M. Kappl, and H.-J. Butt, "Tilt of atomic force microscope cantilevers: effect on spring constant and adhesion measurements." Langmuir 20, 2760–2764 (2004).
11. R.W. Stark, T. Drobek, and W.M. Heckl, "Thermomechanical noise of a free v-shaped cantilever for atomic force microscopy." Ultramicroscopy 86, 207–215 (2001).