An asteroidal companion to the Earth

appeared in Nature, 1997, vol 387, 685-686.

Paul Wiegert and Kimmo Innanen
Department of Physics and Astronomy
York University
4700 Keele Street
North York, Ontario
M3J 1P3 Canada

Seppo Mikkola
Tuorla Observatory
University of Turku
21500 Piikkiö Finland

Near-Earth asteroids (or NEAs) are our closest neighbours in the Solar System-- some of these objects have been known to pass closer to the Earth than the Moon (1). These objects, which range in size from 40 km to a few metres, are important to our understanding of past and present Earth impact rates, and are likely to prove useful as bases and/or mineral sources as humans move into near-Earth space. Here we report that the near-Earth asteroid 3753 (1986 TO) is in an unusual horseshoe-type orbit about that of the Earth. Horseshoe orbits, so named because of their shape in a reference frame which corotates with their accompanying planet, are a well-known feature of the three-body problem (2). However, only one instance of this type of interaction has been observed in nature, involving the Saturnian satellites Janus and Epimetheus (3) , and this system's behaviour is much less intricate than that of 3753. This asteroid is unique: it is an example of a rare type of dynamical relationship, and although it is not a satellite of our planet per se, it is, apart from the Moon, the only known natural companion of the Earth.

Figure 1 presents a view of the inner Solar System projected onto the ecliptic plane in a frame which corotates with the Earth; in this frame, the Earth is stationary. The path of asteroid 3753 over a little more than one year indicated by the line with arrowheads. The path has roughly the shape of a kidney bean, owing simply to the eccentricity of the asteroid's orbit. As the asteroid's orbital period is slightly shorter than that of the Earth, its orbit does not close on itself but rather advances slightly each year; the asteroid thus spirals forward along the orbit of the Earth.

This behaviour is not unusual in itself: what differentiates 3753 from other NEAs is its behaviour as it approaches the Earth. A typical close encounter between the Earth and an NEA results in a large change in the asteroid's orbit, with collision being a distinct, if remote, possibility. However, the dynamical relationship between the Earth and 3753 is such that a much milder interaction occurs. As the asteroid approaches, our planet's gravitational pull acts to increase the asteroid's period to a value slightly greater than one year. As a result, the asteroid begins to fall behind, and hence to move away from, our planet. A possible collision with the Earth is avoided; the closest approach during this leg is indicated by the heavy line A in Figure 1.

When the asteroid eventually approaches the Earth from the other direction, the Earth's gravity will again influence the asteroid's orbit. In this case, however, the asteroid's period decreases to its previous value slightly below that of the Earth; thus the asteroid is again effectively repelled and begins moving away from the Earth. At closest approach on this leg, the edge b of the kidney bean coincides with the heavy line labeled B in Figure 1. The cycle of reversals then goes on to repeat itself. The previous two reversals occurred in approximately AD 1515 and 1900; the next two will occur in 2285 and 2680. The variation in semi-major axis of 3753 over the next two thousand years is presented in Figure 2. The reversals correspond to transitions across the semi-major axis of the Earth (1 AU).

It should be noted that these reversals occur when edge a (not b) approaches the Earth. Owing to the asteroid's orientation and inclination, edge b is not near the Earth's orbit, despite its appearance when projected onto the ecliptic plane. Figure 3 presents a view of the inner Solar System from an ecliptic-on perspective, and the asteroid's high inclination (20 degrees ) is evident. The orientation of the asteroid's orbit allows edge b to overlap the position of the Earth with no danger of collision. The complete orbit of 3753 is thus an ``overlapping horseshoe'' (the outer envelope of which is indicated by the heavy line in Figure 1), a kind which has never been observed before, even in theoretical studies. The asteroid's significant inclination and eccentricity (approx. 0.5) has evidently caused it to escape more intensive scrutiny since its discovery, as they serve effectively to obscure the nature of its trajectory.

The asteroid's orbit is chaotic, with an e-folding time of 150 years, but it remains a near-Earth object in our simulations for time scales of a million years. However, not all of this time is spent as a horseshoe: the asteroid switches between its current orbit and a non-horseshoe orbit with semimajor axis around 1.1 AU on time scales of a few hundred thousand years.

Asteroid 3753 passes from inside to outside the Earth's orbit, but its minimum yearly approach distance is usually quite large. During the closest approaches, which happen only every 385 years, the asteroid passes within 0.1 AU (roughly 40 times the Earth-Moon distance) of our planet, the last such approach having occurred about 100 years ago. Over the next year, the closest approach will be only to within 0.31 AU. The relative proximity of 3753 to the Earth during recent times presumably aided in its discovery by D. Waldron on Oct 10, 1986 at Siding Spring, Australia (4,5) . Asteroid 3753 has an absolute visual magnitude of 15.1 (6), brighter than typical of NEAs, and from which one can estimate a diameter of 5 km (7,8).

Asteroid 3753 crosses the orbits of Venus and Mars as well as the Earth's. Though its orbit does not currently intersect that of any planets, the asteroid's argument of perihelion precesses at a rate of roughly +0.6 degrees per century. As a result, its orbit will intersect that of the Earth in 2750 years, and (if it survives this crossing) that of Venus in about 8000 years. Similarly, the asteroid's orbit intersected Mars' roughly 2500 years ago. These results suggest that the asteroid's current horseshoe orbit may not be stable for arbitrarily long times, unless there is some dynamical ``safety mechanism'' which preserves it against close planetary encounters. At this point, the existence of such a safety mechanism seems unlikely, and yet the very low a priori probability of an object being injected into such an orbit makes a recent origin seem equally unlikely. As for the prediction of the asteroid's future, problematic owing to its short e-folding time, a collision with the Earth seems very improbable. A strong gravitational interaction with Venus in 8000 years seems quite likely, though the possibility of a collision with that planet at that time remains remote.

Methods: The numerical simulations of asteroid 3753 presented here were performed with the Wisdom-Holman (9) integrator, in a model Solar System which included all the planets except Pluto. It should be noted however that the Earth-Moon barycentre was used for the Earth, an approximation which is valid because the Earth-asteroid distance is always much larger than the Earth-Moon distance. The heliocentric orbital elements of 3753 used were: semimajor axis a = 0.99778030 AU, eccentricity e=0.51478431, inclination =19.812285 degrees, longitude of the ascending node=126.373212 degrees , argument of perihelion = 43.640637 degrees , and mean anomaly = 40.048932 degrees , these elements being calculated for epoch JD 2450500.5 and the equinox of J2000.0 (6).

References:

(1) Scotti, J. V., Rabinowitz, P. L., and Marsden, B. G., Near miss of the earth by a small asteroid, Nature, 354, 287-289 (1991).

(2) Szebehely, V., Theory of Orbits, Academic Press: New York (1967).

(3) Synnott, S. P., Peters, C. F., Smith, B. A., and Morabito, L. A., Orbits of the small satellites of Saturn, Science, 212, 191-192 (1981).

(4) Minor Planet Circular 11312, Minor Planet Center, Smithsonian Astrophysical Observatory, Cambridge (1986).

(5) IAU Circulars 4262 and 4266, Central Bureau for Astronomical Telegrams, Smithsonian Astrophysical Observatory, Cambridge (1986).

(6) Bowell, E., The Asteroid Orbital Elements Database, an electronic publication available at
ftp://ftp.lowell.edu/pub/elgb/astorb.html (1997).

(7) Bowell, E., Hapke, B., Domingue, D., Lumme, K., Peltoniemi, J. and Harris, A. W., Application of photometric models to asteroids, in Asteroids II, Binzel, R. P. et al. (eds.), The University of Arizona Press, Tucson, pp. 524-556 (1989).

(8) Rabinowitz, D. L., The size distribution of earth-approaching asteroids, Astrophys. J., 407, 412-427 (1993).

(9) Wisdom, J. and Holman, M., Symplectic maps for the n-body problem, Astron. J, 102, 2022-2029 (1991).

Acknowledgements: We would like to thank Matt Holman for the use of his numerical code, and the referees for their helpful comments. This work has been supported in part by the National Sciences and Engineering Research Council of Canada. Correspondence and requests for material to P.A.W., (wiegert@yorku.ca).

 
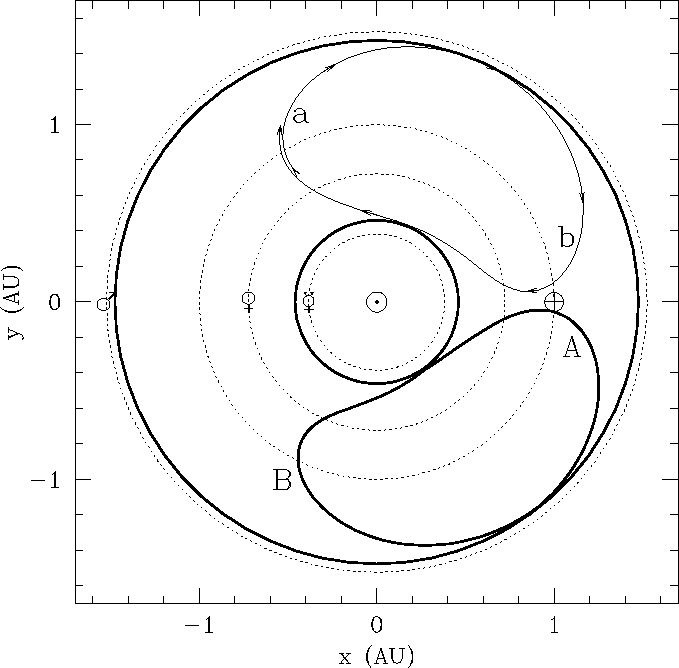
Figure 1: A view of the inner Solar System projected onto the ecliptic plane in a frame which corotates with the Earth; in this frame, the Earth is stationary and is located at the crossed circle. The average distances of the planets Mercury, Venus, Earth and Mars from the Sun are indicated by the dotted circles. The path of asteroid 3753 over slightly more than a year, beginning approximately in AD 2000, is shown by the line with arrowheads. The extremes of the horseshoe are indicated by the heavy lines. One extreme occurs when a coincides with A, the other when b coincides with B.

 
Figure: The heliocentric semimajor axis a of asteroid 3753 (1986 TO) over the next two thousand years, in astronomical units (AU). Transitions from a >1 AU to a < 1 AU or vice-versa correspond to reversals of the asteroid's direction within the horseshoe.

 
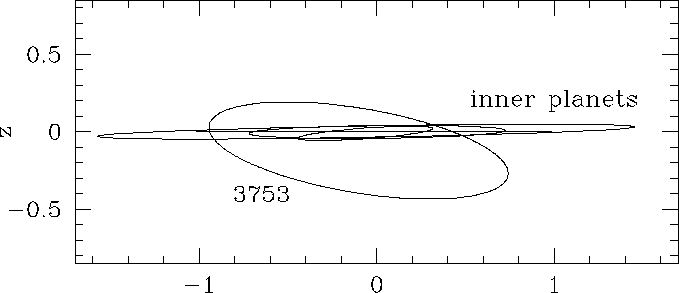
Figure 3: The orbits of the inner planets Mercury, Venus, Earth and Mars, along with that of asteroid 3753 when seen from the direction of the vernal equinox. The Sun is at the origin, and the units are astronomical units.