|
The ability of the atomic force microscope (AFM) to measure forces at the pico-Newton scale with
nanometer lateral resolution is well suited to the study of nanomaterials such as carbon nanotubes.
Since nanomaterials with high aspect ratios are used as a reinforcing phase in composite materials,
it is important to understand their mechanical properties. We have been using the AFM to study two
such materials: bacterial cellulose (BC), which can be isolated as bundles (25–75 nm across) of
individual fibres, and multiwalled carbon nanotubes (MWCNTs), with typical diameters of 10–40nm.
|
|

AFM image of bacterial cellulose fibres suspended across a grating
|
| | | |
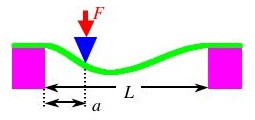
Schematic of an AFM tip deflecting a suspended beam
|
|
In our experiments, we use the AFM to apply known forces at various positions along a fibre or
tube suspended across a known gap while simultaneously measuring the resulting deflection. By
comparing the "compliance profile" thus obtained with expectations based on the elastic
theory of beams, we are able to extract elastic parameters such as the Young's modulus and shear
modulus.
|
| | | |
| For bacterial cellulose, we find that the Young's modulus is 78 ± 17 GPa (roughly the same as for
aluminum) and does not vary with fibre diameter (as would be expected for solid tubes of isotropic material).
Since the AFM has no way to measure the inner diameter of carbon nanotubes, a
reliable estimate of their intrinsic mechanical properties is more difficult to obtain. We see that
the "bending modulus" (which is due to both the Young's modulus and shear modulus, as well as
the geometry) increases with decreasing outer tube diameter in a manner consistent with the
known structure of a hollow tube consisting of rigid shells with weak van der Waals interactions
between shells. It is likely that the weak bonds between shells, as well as defects within the tube,
prevent the elastic properties from achieving the moduli of several TPa predicted by some theoretical
studies.
|
|
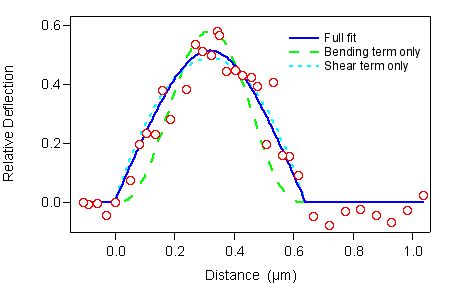
Deflection of a carbon nanotube relative to the deflection of the AFM
cantilever as a function of position across the gap. The curves indicate fits to various
elastic models.
|


